term 1 xii maths

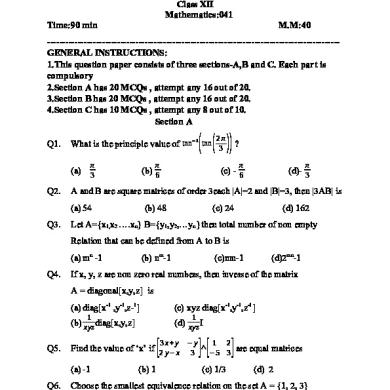
VIKAS BHARATI PUBLIC SCHOOL Preboard Examination (Session 2021-22) Class XII Mathematics:041 Time:90 min

M.M:40

-----------------------------------------------------------------------------------------------GENERAL INSTRUCTIONS: 1.This question paper consists of three sections-A,B and C. Each part is compulsory 2.Section A has 20 MCQs , attempt any 16 out of 20. 3.Section B has 20 MCQs , attempt any 16 out of 20. 4.Section C has 10 MCQs , attempt any 8 out of 10. Section A Q1.

2π −1 What is the principle value of tan tan 3

( ( )) ?

π 3

(a) Q2.

π

π

(c) - 6

(d)- 3

A and B are square matrices of order 3each |A|=2 and |B|=3, then |3AB| is (a) 54

Q3.

π

(b) 6

(b) 48

(c) 24

(d) 162

Let A={x1,x2 ….xn} B={y1,y2,…yn }then total number of non empty Relation that can be defined from A to B is (a) mn -1

Q4.

(b) nm-1

(d)2mn-1

(c)mn-1

If x, y, z are non zero real numbers, then inverse of the matrix A = diagonal[x,y,z] is (a) diag[x-1 ,y-1,z-1] 1

1

(b) xyz diag[x,y,z] Q5.

(d) xyz I

[ 3 x+ y

Find the value of ‘x’ if 2 y −x (a) -1

Q6.

(c) xyz diag[x-1,y-1,z-1]

−y ∧ 1 2 are equal matrices 3 −5 3

][

(b) 1

]

(c) 1/3

(d) 2

Choose the smallest equivalence relation on the set A = {1, 2, 3} (a){(1,2) (2,1) (1,1)(2,2)} (b) {(1,1)(2,2)(3,3)} (c) {(3,3)(2,2)(1,1)(2,3)(3,2)} (d) {(1,2)(2,1)(3,1)(1,3)(1,1)(3,3)} 1

Q7.

Find the interval for the function f(x)=4x3 -18x2+27x-7 is increasing. 3

(a)f(x) is increasing on R

(b) f(x) is increasing on ( 2 ,∞ ) 3

(c)f(x) is increasing on (- ∞, 2 ) (d)none of these 3

Q8.

3

then adj A is

[ ] (c) [−59 −32 ] (d) 13 [59 32] 1−x Differentiate tan ( √ w.r.t cos ( 2 x √1−x ) x )

(a) 3 1 −5 2

[

Q9.

−1 2 3

[ ]

If |A|=3 and A-1= −5

1 9 3

]

(b) 3 5 2

2

-1

1

1

(a) 2

2

−1

(b) - 2

(c) 1

(d) none of these

0 2 b −2 Q10. Matrix A= 3 1 3 is given to be symmetric , find values of ‘a’ . 3 a 3 −1

[

]

3

−2

(a) 3

2

(b) 2

−3

(c) 3

(d) 2

Q11. For what values of k, the system of linear equations has a unique Solution?

x+y+z=2, 2x+y-z=3, 3x+2y+kz=4

(a)k=1

(b) k = -1

(c) k∈ R

(d) k∈R-{0}

[3 5 ]

Q12. If A = 7 9 is written as A=P+Q where P is symmetric matrix and Q is a skew symmetric then write the matrix P. (a)

[−63 −69 ]

[3 6 ]

(b) 6 9

[−3 −69 ]

(c) 6

[3

6

(d) −6 −9

]

Q13. The domain of √ x−1 +√ 8− x is (a) [1,8]

(b) [-8,8]

(c) [1,8)

(d) (1,8)

Q14. If f:R→R be given by f(x)=3x then (a)f is one one but not onto (b) f is many one and onto 2

(c) f is one one and onto

(d) f is neither one one nor onto.

Q15. What is the maximum value of sinx + cosx? (a) 2

(b) √ 2

(c) 1

(d) none of these

Q16. Let R = {(a, a) (b, b) (c, c) (a, b)} be a relation on set A = {a, b, c}. Then R is (a) Identity relation

(b) Equivalence relation

(c) Symmetric relation

(d) Reflexive relation

Q17. For set A = {1, 2, 3} define a relation R in set A as follows R = {(1, 1) (2, 2) (3, 3) (1, 3)}. Write the ordered pairs to be added to R to make it the smallest equivalence relation. (a) (2, 3)

(b) (3, 2)

(c) (3, 1)

(d) (3, 1) (2, 3)

Q18. If A = {1,2, 3, ….. 12} given by R = {(a, b): |a−b| is divisible by 3} is an equivalence relation, then all elements related to the element 1 is (a) {1, 4, 7} (b) {1, 7, 3, 4}

(c) {1, 4, 7, 10} (d) {4, 6, 7, 8}

π

Q19. If tan-1x= 10 for some x ∈ R, then the value of cot-1x is π

(a) 5

2π

6π

(b) 5

(c) 10

8π

(d) 10

Q20. Corner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8) And (0,5). Let F= 4x+6y be the objective function then the minimum Value of F occurs at (a) (0,2) only (b) (3,0) only (c) the mid point of the line segment joining the points (0,2) and (3,0) only (d) Any point on the line segment joining the points (0,2) and (3,0). Section B

(π )

Q21. The derivative of tan 2 −x is equal to

3

(π )

(a) Sec2 2 −x

(b)-cosec2x

(π ) (π )

(c) cosec2x

(d) sec 2 −x tan 2 −x

dy

Q22. If xpyq = (x+y)p+q then dx is x

y

(a) y

x

(b) x

y

(c) x+ y

(d) x+ y

Q23. Which of the following is the principle value branch of cosec-1x ? π

π

(a) ¿ , 2 )

π π

(b) [0, π ]-{ 2 }

π π

(c) [- 2 , 2 ] (d) [- 2 , 2 ]-{0}

Q24. Let A={1,2,3,4} and B = {1,2,3,4,5,6}then number of one to one functions from A to B is a) 0

(b) 60

(c) 120 (d) 360

−1 y Q25. Sec{ tan ( 3 )} is equal to :

9+ y 2 √ (a) 9

9+ y 2 √ (b)

(c)

3

3

√ 9+ y

(d)

2

9

√ 9+ y 2

[ 2] [−1] [ 10 ]

Q26. If x 3 +y 1 = 5 then the values of x and y are : (a) x=3, y=7

(b) x=2 , y=-6 (c) x=5, y=2 (d) x=3,y=-4

Q27. The point at which the maximum value of Z= x+y subject to the Constraits : x +2 y ≤ 70 ,2 x+ y ≤ 80 , x , y ≥0 Is obtained at (a) (30,25)

(b) (20,35)

(c) (30,20) (d) None of these

Q28. The equation of tangent to the curve y = 2x2-2x+1 at the point (1,3) is (a) Y-2x-1=0 (b) y+2x-3=0

(c) 2y +4x+3 = 0 (d) 2y-x+4=0

Q29. The derivative of cos−1 ( 2 x 2−1 ) with respect to cos-1x is (a) 2 Q30. lim ¿x → 0 (a) 2

(b)

2

−1 2 √ 1−x

(c) x

2

(d) None of these

e x −e−x ¿ is equal to x

(b) 0

(c) 1

(d) None of these

4

4−x 2 Q31. If function f(x) = is 4 x−x 3

(a) Discontinuous at only one point (b) Discontinuous at exactly two points (c) Discontinuous at exactly three points (d) None of these Q32. The corner points of the feasible region determined by the following system of linear inequalities. 2x + y ≤ 10, x + 3y ≤ 15, x, y ≥ 0 are (0, 0)(5, 0)(3, 4) and (0, 5) Let Z = px + qy where p, q > 0 conditions on p and q, so that the maximum of Z occurs at both (3, 4) and (0, 5) is (a) p = q

(b) p = 2q

(c) p = 3q

(d) q = 3p

Q33. Let R be the relation in the set N, given by R={(a,b):a=b-2,b>6} then (a) (8,7) ∈ R

(b) (6, 8) ∈ R

(c) (3, 8) ∈ R

(d) (2, 4) ∈ R

Q34. If A is a 3x3 invertible matrix then what will be the value of k if det(A-1) = (detA)k ? (a) K=1

(b)K=-1

(c)K=2

(d)K=-2

Q35. Find two positive numbers x and y such that x + y = 60 and xy3 is maxima. (a) 15, 45

(b) 13, 47

(c) 10, 50

(d) 20, 40

Q36. The normal at the (1, 1) on the curve 2y + x2 = 3 is (a) x + y = 0 (b) x + y + 1 = 0

(c) x – y = 0 (d) 2x + y = 1

Q37 The function f(x) = x3 – 3x is (a) Increasing in (-∞ , -1) ∪ [1, ∞ ) and decreasing in (-1, 1) (b) Decreasing in (-∞ , -1) ∪ [1, ∞ ) and increasing in (-1, 1) (c) Increasing in (0, ∞ ) and decreasing in (-∞ , 0) (d) Decreasing in (0, ∞ ) and increasing in (-∞ , 0) Q38 The minimum value of xlogx is

5

1

(a) e

−1

(b) e

(c) e

(d) none of these

x 2 Q39 The local minimum of the point f(x) = 2 + x is

(a) at x = 2

(b) at x = -2

(c) at x = 0

(d) at x = 1

dy −1 sinx+cosx Q40. If y = tan cosx−sin x then dx is

(

1

)

π

(a) 2

(b) 4

(c) 0

(d) 1

Q41. If A is a square matrix such that |A| = 5. Write the value of |AAT| (a) 20

(b) 12

(c) 25

(d) none of these

Q42. For what value of k is the given function continuous at x = 0. sin 5 x +cos x , if x ≠ 0 f(x) = 3 x x=0 k,

{

3

(a) 8

8

5

(b) 3

8

(c) 3

(d) - 3

The following questions consist of two statements-Assertion (A) and Reason(R) . Answer these questions selecting the appropriate option given below : (a) Both A and R are true and R is the correct explanation for A. (b)Both A and R are true and R is not the correct explanation for A. (c) A is true but R is false. (d)A is False but R is true.

{ x+1 , x <2

Q43. Assertion(A): If f(x) = 2 x−1 , x ≥ 2 then f´(x) does not exist Reason(R): f(x) is continuous at x = 2 x

d ( xx ) Q44. Assertion(A): = x x . x(1+2logx) dx x

2

2

Reason(R): (xx)x = x x = e x logx Q45. Assertion(A): In an LPP, the maximum value of the objective function Z = px + qy is always finite Reason(R): Every LPP has unique optimal solution 6

Case Study These days Chinese and Indian troops are engaged in aggressive melee, faceoffs skirmishes at locations near the disputed Pangong lake in Ladakh. One day a helicopter of enemy is flying along the curve represented by y = x2 + 7. A soldier placed at (3, 7) wants to shoot down the helicopter when it is nearest to him. Based on the above information answer the following questions: Q46. If (x1, y1) represents the position of helicopter on the curve y = x2 + 7, when the distance D from soldier placed at S(3, 7) is minimum the the relation between (x1, y1) is (a) x1 = y12 + 7

(b) y1 = x12 + 7

(c) y1+x12 = 7

(d) x1+y12 = 7

Q47. The distance ‘D’ expressed as a function of x1 is (a) D = x12- 6x1+ x14 (b) D2 = x12- 6x1+ 9 + x14

(c) D = x12- 6x1+ 9 + x14 (d) D2 = x12+ 6x1 – 9 + x14

Q48. The soldier at X wants to know when the enemy helicopter is nearest to soldier, then the value of y1 should be (a) 4

(b) 3

(c) 8

(d) 5

Q49. When the enemy helicopter is nearest to soldier, then the value of D should be (a) 4 units

(b) 5 units

(c) √ 5 units

(d) √ 7 units

Q50. The nearest position of helicopter from soldier is (a) (1, √ 5)

(b) (1, 8)

(c) (1, 7)

7

(d) (1, √ 7)