MAT150_TPR2-convertido (1)

MAT 150 ( CALCULO II)

TRABAJO PRACTICO # 2 ( Derivadas Parciales) Semestre II-2020

Ing. Agustín Camacho

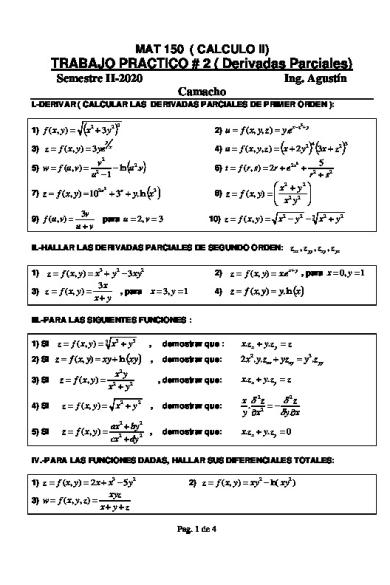
I.-DERIVAR ( CALCULAR LAS DERIVADAS PARCIALES DE PRIMER ORDEN ): 1) f ( x, y ) =

(x

2

+ 3y2

3) z = f ( x, y ) = 3 ye 5) w = f (u, v) =

)

3

2) u = f ( x, y, z) = y.e x−z + y 5

(

y

4) u = f ( x, y, z ) = x + 2 y 2

x

( )

v2 − ln u 2 .v 2 u −1

9) f (u , v) =

3v u+v

4

2 3

5 r + s2  x2 + y2  8) z = f ( x, y ) =  2 2   x y  6) t = f (r , s ) = 2r + e 2 s + 3

( )

7) z = f ( x, y) = 103 x + 3x + y.ln x3 2

) (3x + z )

10) z = f ( x, y ) =

para u = 2, v = 3

2

x2 − y 2 − 3 x2 + y 2

II.-HALLAR LAS DERIVADAS PARCIALES DE SEGUNDO ORDEN: z xx , z yy , z xy , z yx

z = f ( x, y) = x3 + y 2 − 3xy2 3x 3) z = f ( x, y ) = , para x = 3, y = 1 x+ y 1)

2)

z = f ( x, y) = x.e x+ y , para x = 0, y = 1

4)

z = f ( x, y) = y. ln (x)

III.-PARA LAS SIGUIENTES FUNCIONES : 1) Si

z = f ( x, y ) = 3 x 3 + y 3

2) Si z = f ( x, y) = xy + ln (xy)

,

demostrar que :

x.z x + y.z y = z

,

demostrar que:

2 x2 . y.z xx + yzxy = y3.z yy

x2 y x2 + y 2

x.z x + y.z y = z

3) Si

z = f ( x, y) =

4) Si

z = f ( x, y ) = x 2 + y 2

,

demostrar que:

x  2z  2z . 2 =− y x y.x

5) Si

ax 2 + by 2 , z = f ( x, y) = 2 cx + dy 2

demostrar que:

x.z x + y.z y = 0

, demostrar que:

IV.-PARA LAS FUNCIONES DADAS, HALLAR SUS DIFERENCIALES TOTALES: 1) z = f ( x, y) = 2 x + x3 − 5 y 2 3) w = f ( x, y, z ) =

2) z = f ( x, y) = xy2 − ln( xy2 )

xyz x+ y+z

Pag. 1 de 4

V.-HALLAR LAS DERIVADAS TOTALES (REGLA DE LA CADENA) : 1) z = f ( x, y) = xy − y 2

Si

x = f (r , s ) = r 3 + 2

s , r

(

)

y = f (r, s) = er + 5r. s3 + 3 2

2

 2   Si x = f (u) = ln (u 2 + 1) , 2) z = f ( x, y ) =  y 2 − 2 2  x +y   2 3 x y 3) z = f ( x, y ) = Si x = f (r , s ) = 3 , r + s3 x+ y

y = f (u ) =

5 u +3 3

(

)(

y = f (r , s) = 3s 2 − 2 . r 3 + 4

)

VI.-PARA LAS SIGUIENTES FUNCIONES IMPLICITAS:

( )

1) Si: ln xz3 + x 2 z 5 − 3 y + 4 z = 0 , Hallar

 y2  2) Si: 2 x. y + ln    x  3) Si: f ( x, y, z ) = 0 . 2

,

x

( yz ) + 2 = 0

dy dx

Demostrar que:

4) Si: ax 2 + by 2 + cz 2 = 0 , 5) Si: xy −

Hallar

z z ,  x x

,

6) Si: e x + e y + e z − e xyz = 0

Hallar z x

,

zy

Hallar z x

,

zy

Hallar z x

,

zy

x y . y z .z x = −1

VII.-PARA LAS FUNCIONES DADAS SIN RESTRICCIONES DETERMINAR MÁXIMOS, MINIMOS RELETIVOS, PUNTOS DE SILLA ( SI LOS HAY): a) z = f ( x, y) = xy − ln( x 2 − y 2 )

b) z = f ( x, y) = xy − 2 y 2

c) z = f ( x, y ) = xy + x − y

c) z = f ( x, y ) = 2 x 2 − 2 xy + y 2 + 5x − 3 y

d) z = f ( x, y ) = x 2 − y 2 − 2 x − 2 x + 4 y + 6

e) z = f ( x, y) = 4 x + 2 y − x 2 + xy − y 2

VIII.-PARA LAS SIGUIENTES FUNCIONES HALLAR MÁXIMOS Y MINIMOS SUJETOS A LAS RESTRICCIONES DADAS: a)

z = f ( x, y) = x 2 − 10 y 2

Si:

x − y = 18

b)

z = f ( x, y ) = 3x 2 + 4 y 2 − xy ,

si

2 x + y = 21

c)

z = f ( x, y ) = x. y ,

si

x2 + y2 =1

d) z = f ( x, y ) = 4 x 2 + 5 y 2 − 6 y ,

si

x + 2 y  18 , Hallar el mínimo

z = f ( x, y ) = 3 x 2 + 3 y 2 ,

si

x + y  10 , Hallar el mínimo

e)

Pag. 2 de 4

IX.- DETERMINAR SI CADA UNA DE LAS SIGUIENTES FUNCIONES ES HOMOGÉNEA. PARA LAS QUE SEAN HOMOGÉNEAS DETERMINAR EL GRADO Y DEMOSTRAR EL TEOREMA DE EULER: a)

z = f ( x, y) = 2 x 3 + 5x 2 y + 3 y 3

c)

z = f ( x, y ) =

x 4 + x 2 y 2 + 2x3 y − y 4 x2

b)

z = f ( x, y) = 2e x + 2e y

d)

z = f ( x, y ) =

x5 y + y 6 x3

X.- RESOLVER LOS SIGUIENTES EJERCICIOS DE APLICACIÓN: 1) Para cada uno de los siguientes pares de funciones de demanda, determinar las cuatro demandas marginales parciales. x = f ( p, q) = 20 − 2 p − q , y = f ( p, q) = 9 − p − 2q a) b)

x = f ( p, q ) =

4 p2q

,

y = f ( p, q ) =

16 pq 2

2) Para cada una de las siguientes funciones de producción, hallar las productividades marginales:

1 1 − x y

a)

z = f ( x, y ) = 25 −

b)

z = f ( x, y ) = 5xy − 2 x 2 − 2 y 2

c)

16 z − z − 80( x − 5) + 2( y − 4 ) = 0

d)

16 z 3 − z − 6 x − 24 y + x 2 + 4 y 2 + 50 = 0

2

, en x = 1 , y = 1 , en x = 1 , y = 1

2

3) Un editor tiene que distribuir $ 60.000 para gastar en desarrollo y promoción de un nuevo libro. Se estima que si se gastan en desarrollo x miles de dólares y en promoción y miles 3

de dólares, se venderán aproximadamente 20.x 2 . y ejemplares del libro. Cuanto dinero debe dedicar el autor a desarrollo y cuanto a promoción con el objeto de maximizar sus ventas? ( Respuesta: $ 36.000 en desarrollo y $ 24.000 en promoción ) 4) Hallar las cantidades “x” y “y” y los precios “p” y “q” que maximicen la ganancia y hallar las máximas ganancias para cada uno de los siguientes conjuntos de funciones de demanda y funciones de costo conjunto para dos mercancias: x = f ( p, q ) = 1 − p + 2 q , y = f ( p, q) = 11 + p − 3q , C = f ( x, y ) = 4 x + y a) b)

p = f ( x) = 40 − 2 x 2 ,

q = f ( y ) = 12 − 3 y , C = f ( x, y ) = 8 + 4 x + 3 y

5) El costo de producción “C” ,como una función de las cantidades producidas: “x” y “y” de dos tipos de artículos está dado por: ¿Qué cantidades de C = f ( x, y ) = 6 x 2 + 3 y 2 los dos artículos deben producirse para minimizar el costo si: a) x + y = 18 b) x + y  18

Pag. 3 de 4

6) La producción “Z” como función de los insumos “x” y “y” ,está dada por: Hallar las cantidades de “x” y de “y” que maximizan la Z = f ( x, y) = x 2 + 5xy − 4 y 2 2 x + 3 y = 74 producción si: a) 2 x + 3 y  74 b) 7) El número de fallas “N” como función de los números “x” y “y” de cambios de dos partes de una máquina está dado por la función : N = f ( x, y ) = 3x 2 + y 2 + 2 xy − 22 x + 6 Para minimizar las fallas, ¿Qué números de cambios deben hacerse de cada parte si : 2x = y ? 8) Una firma encuentra que si gasta “x” pesos en desarrollo de productos y “y” pesos en propaganda, entonces sus utilidades anuales se incrementan en :

f ( x, y ) = 3 x + 2 y −

1 2 1 2 y − x 100 200

a)Hallar el incremento máximo de las utilidades anuales que puede obtener dicha firma. b) Si la firma decide gastar solamente en desarrollo de productos, en cuanto se incrementan sus utilidades ? 9) La función de producción z = f ( x, y) = 84 x + 96 y − 3x 2 − 4 y 2 , donde x y y son dos factores de producción, corresponde a la fabricación de un producto. Hallar la producción máxima. 10) Una fábrica produce dos artículos A y B relacionados de cantidades x y y respectivamente. Sus demandas son p = 72 − x , q = 42 − 3 y , donde p y q son sus respectivos precios . El total que produce la fábrica es de 56 unidades. Hallar el máximo ingreso conjunto.

Pag. 4 de 4